This , so the legend has it , is a temple in Benares . They say that when the great lord Brahma created the world he put up 3 diamond sticks , mounted on a brass plate , beneath the dome that marks the center of the world . Upon one of the sticks he placed 64 gold discs .The biggest at the bottom , all decreasing in size , with the smallest at the top . The temple priests have the tasks of transposing the discs from one stick to other , using the third as an aid . They work day and night , but must transpose only one disc at a time and must not put a bigger disc on top of a smaller one . When their task is complete , the legend says , the world will disappear in a clap of thunder .
Now the total number of transpositions needed to shift the 64 disc is 2 raised to 64 minus 1 , or 18446744073709551615 . If every transpositions takes a second it will take 500000 million years to get the job done .
Origin of the Game
The Tower of Brahma, or the Tower of Hanoi, as it is sometimes called, was the invention of the French mathematician Edouard Lucas and was sold as a toy in France in 1883.
The legend of the Temple of Benares is also his invention.
This is a great game to get young children (5- or 6-year old) started and on a par with Hop Over.
This is a great game to get young children (5- or 6-year old) started and on a par with Hop Over.
Description
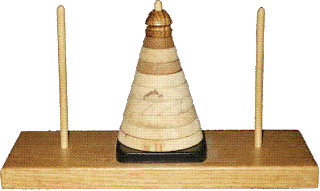
The original device consists of a base supporting 3 vertical pegs placed in a straight line, with a conical tower of 8 to 10 disks of the same or indeterminate color.
The original device consists of a base supporting 3 vertical pegs placed in a straight line, with a conical tower of 8 to 10 disks of the same or indeterminate color.
The Rules
The tower of disks must be transported from the left peg to the right peg, (a) moving only 1 disk at a time, and (b) never placing a disk on a smaller one than itself, thus always conserving a tower, cone-like, shape. The middle peg is evidently temporarily employed in the process. The game is fiendishly difficult with this model.
The tower of disks must be transported from the left peg to the right peg, (a) moving only 1 disk at a time, and (b) never placing a disk on a smaller one than itself, thus always conserving a tower, cone-like, shape. The middle peg is evidently temporarily employed in the process. The game is fiendishly difficult with this model.
Mathematics
To see the mathematics involved in the game.
To see the mathematics involved in the game.
Several things can be done to help the child observe and understandthe mathematical regularities of this game -(a) Place the pegs preferably in triangular rather than linear formto clearly observe the direction of the movement of each disk.(b) Color the odd-numbered disks one color and even-numbered disks another color,to clearly observe the alternation of colors at all times,disks of the same color NEVER touching each other.A detailed pedagogical approach is seen later in the "card model".



No comments:
Post a Comment